meetingqosa.blogg.se

The density of the ethylene glycol is constant.

If the depth of the liquid is 0.8 m, what is the pressure at the bottom of the tank? Approach: Use the equation for pressure as a function of depth in an incompressible fluid: P = P∞ + ρ gh. The air space at the top of the closed vat is maintained at 110 kPa. ⎠ ⎝ 1000 Pa ⎠ ⎦ ⎣ P = 120 kPa 4-4 Answer A vat in a chemical processing plant contains liquid ethylene glycol at 20oC. Solution: At an assumed temperature of 20✬, the density of mercury (from Table B-6) is ρ Hg = 13,579 kg/m3 The pressure in an incompressible fluid as a function of depth is P = Patm + ρ Hg gh ⎡ ⎛ 0.3048 m ⎞ ⎛ 1 kPa ⎞ ⎤ P = 100 kPa + ⎢(13,579 kg/m3 )( 9.8 m/s 2 ) ( 6in.) ⎜ ⎟⎜ ⎟⎥ ⎝ 12 in. The mercury is at room temperature (20✬). If atmospheric pressure is 100 kPa, what pressure is the manometer reading (in kPa)? Approach: Use the equation for pressure as a function of depth in an incompressible fluid: P = Patm + ρ gh. ⎠ ⎠ Answer 4-3 In a manometer containing liquid mercury, the height is read as 6 in. Solution: At an assumed temperature of 70✯, the density of water (from Table B-6) is ρ H 2O = 62.2 lbm/ft 3 The pressure in an incompressible fluid as a function of depth is P = Patm + ρ gh ⎛ ⎜ 1 lbf lbf ⎛ lbm ⎞⎛ ft ⎞ = 14.7 2 + ⎜ 62.2 3 ⎟⎜ 32.2 2 ⎟ ( 725ft ) ⎜ in.

Approach: Use the equation for pressure as a function of depth in an incompressible fluid: P = Patm + ρ gh. Assuming atmospheric pressure is 14.7 lbf/in.2, calculate the pressure in the reservoir at the base of the dam. ⎠ ⎦ ⎣ Answer P = 19.0 lbf in.2 4-2 Hoover dam stands at a height of 725 ft above the Colorado river. Solution: At an assumed temperature of 70✯, the density of water (from Table B-6) is ρ H 2O = 62.2 lbm/ft 3 The pressure in an incompressible fluid as a function of depth is P = Patm + ρ gh ⎡ ⎛ ⎞ ⎛ 1ft 2 ⎞ ⎤ 1lbf = 14.7 lbf in.2 + ⎢( 62.2 lbm/ft 3 )( 32.2 ft/s 2 ) (10 ft ) ⎜ 2 ⎟⎜ 2 ⎟⎥ ⎝ 32.2 lbm ⋅ ft/s ⎠⎝ 144in.

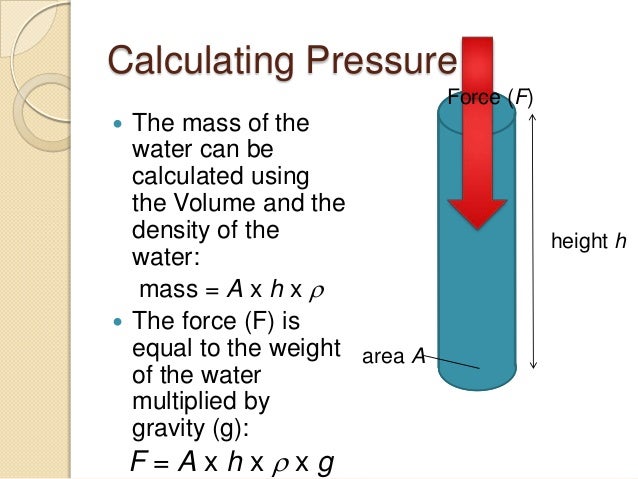
4-1 If atmospheric pressure is 14.7 lbf/in.2, what is the pressure at a depth of 10 ft of water? Approach: Use the equation for pressure as a function of depth in an incompressible fluid: P = Patm + ρ gh.